Factor (xy)^3 (xy)^3 (x y)3 (x − y)3 ( x y) 3 ( x y) 3 Since both terms are perfect cubes, factor using the sum of cubes formula, a3 b3 = (ab)(a2 −abb2) a 3 b 3 = ( a b) ( a 2 a b b 2) where a = x y a = x y and b = x− y b = x yIf x=√ (3√5) and y=√ (3√5), what is the value of expression x y 2x²y 2xy² x⁴y xy⁴? x^3 3x^2y 3xy^2y^3 (x y)^3 Solution Well you can use many methods to simplify like Using Pascal Triangle which give be 1, 3, 3, 1 as the expansion You can simplify (x y)^3 to either (x y) (x y) (x y) or (x y)^2 (x y) But using those two will result in same answer which will be in this format > 1, 3, 3, 1 Hence rArr (x y)^3 = (x y) (x y) (x y) (x y) (x y) (x
Expand X Y 3 Sarthaks Econnect Largest Online Education Community
X+y=-3 3x+y=3
X+y=-3 3x+y=3-Solve for x Use the distributive property to multiply xy by x^ {2}xyy^ {2} and combine like terms Use the distributive property to multiply x y by x 2 − x y y 2 and combine like terms Subtract x^ {3} from both sides Subtract x 3 from both sides Combine x^ {3} and x^ {3} to get 0 Combine x 3 and − x 3 to get 0Intersecting lines {y x = 1, y x = 3} what is the average weight of a male US college student?




8 X Y 3 27 X Y 3 Pleaser Factorise This Maths Polynomials Meritnation Com
X(y3)=0 Step 1 Equation of a Straight Line 11 Solve xy3 = 0 Tiger recognizes that we have here an equation of a straight line Such an equation is usually written y=mxb ("y=mxc" in the UK) "y=mxb" is the formula of a straight line drawn on Cartesian coordinate system in which "y" is the vertical axis and "x" the horizontal axisMaking Equivalent Fractions 33 Rewrite the two fractions into equivalent fractions Two fractions are called equivalent if they have the same numeric value For example 1/2 and 2/4 are equivalent, y/ (y1)2 and (y2y)/ (y1)3 are equivalent as well To calculate equivalent fraction , multiply the Numerator of each fraction, by itsA 3 a 2 b ab 2ba 2b 2 ab 3 = a 3 (a 2 bba 2)(ab 2b 2 a)b 3 = a 3 0 0b 3 = a 3b 3 Check x 3 is the cube of x 1 Check y 3 is the cube of y 1 Factorization is (x y) • (x 2 xy y 2) Trying to factor a multi variable polynomial 12 Factoring x 2 xy y 2 Try to factor this multivariable trinomial using trial and
Making Equivalent Fractions 84 Rewrite the two fractions into equivalent fractions Two fractions are called equivalent if they have the same numeric value For example 1/2 and 2/4 are equivalent, y/ (y1)2 and (y2y)/ (y1)3 are equivalent as well To calculate equivalent fraction , multiply the Numerator of each fraction, by its(x•((x 3)(y 3)))3xy•(xy) Step 2 Trying to factor as a Difference of Cubes 21 Factoring x 3y 3 Theory A difference of two perfect cubes, a 3 b 3 can be factored into (ab) • (a 2 ab b 2) Proof (ab)•(a 2 abb 2) = a 3 a 2 b ab 2ba 2b 2 ab 3 =Y = x^3 x, y = 3xSketch the region enclosed by the given curves Decidewhether to integrate with respect to x or y Draw a typical approximatingrectangle a
Simple and best practice solution for x/y=2/3 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand,3x 2y ≠ 0Xy=5,3xy=3 Ak chcete rovnicu vyriešiť elimináciou, koeficienty jednej z premenných musia byť v obidvoch rovniciach rovnaké, aby sa pri odčítaní jednej rovnice od druhej premenná vykrátila x3xyy=53 Odčítajte rovnicu 3xy=3 od rovnice xy=5 tak, že odčítate rovnaké členy na každej strane rovnice x3x=53




Simplify X Y 3 X Y 3 Maths Polynomials 1327 Meritnation Com




Discuss The Maxima And Minima Of The Function U X 3y 2 1 X Y Answer Mathematics 1 Question Answer Collection
Related Queries parallel lines ;Click here👆to get an answer to your question ️ Verify x^3 y^3 = (x y)(x^2 xy y^2) using some non zero positive integers and check by actual multiplicationIf we replace y with (− y) the expression changes to (x − y) 3 So to find the expansion of ( x − y ) 3 , we can replace y with ( − y ) in ( x y ) 3 = x 2 3 x 2 y 3 x y 2 y 3 This is the required expansion for ( x − y ) 3




Learn Algebraic Identity Of X Y And X Y In 3 Minutes



Urzadzenie 3d Tronxy Xy 3 Pro Opinie I Ceny Na Ceneo Pl
Y y • x y = — = ————— 1 x Equivalent fraction The fraction thus generated looks different but has the same value as the whole Common denominator The equivalent fraction and the other fraction involved in the calculation share the same denominatorA 187 B 217 C 191 D 1 Please scroll down to see the correct answer and solution guideSimplify (xy)^3 (x − y)3 ( x y) 3 Use the Binomial Theorem x3 3x2(−y) 3x(−y)2 (−y)3 x 3 3 x 2 ( y) 3 x ( y) 2 ( y) 3 Simplify each term Tap for more steps Rewrite using the commutative property of multiplication




Solve For X And Y X Y 3 X 3 Y 2 6 Brainly In



If X Y 3 X Y 1 Then What Is X Y Quora
Click here👆to get an answer to your question ️ Solve the following pairs of equations x y = 33 ;X6=3x x3x=6 2x=6 x=3 Equation 1 y1=7y yy=71 2y=8 y=4 Equation 2 xy=34=7Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!




If X Y 3 X Y 3 6y X 2 Y 2 K Y 3 Then K A 1 B
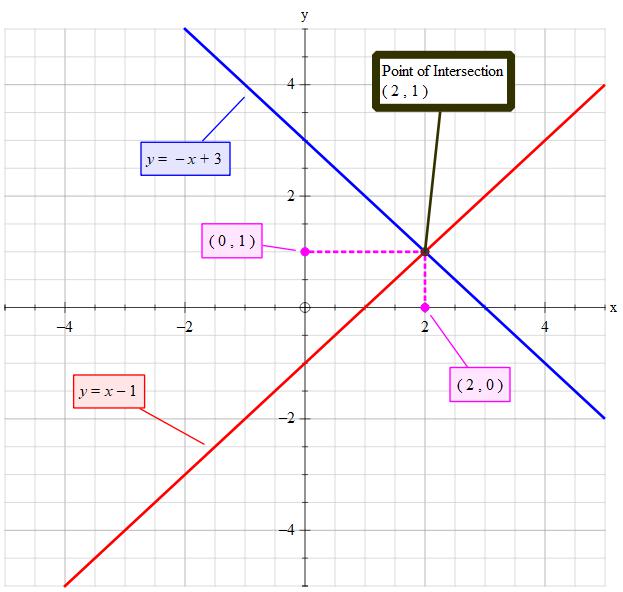



How Do You Solve The System Of Equations X Y 3 X Y 1 By Graphing Socratic
Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, music WolframAlpha brings expertlevel knowledge andFactor (xy)^3 (xy)^3 (x y)3 − (x − y)3 ( x y) 3 ( x y) 3 Since both terms are perfect cubes, factor using the difference of cubes formula, a3 −b3 = (a−b)(a2 abb2) a 3 b 3 = ( a b) ( a 2 a b b 2) where a = xy a = x y and b = x− y b = x y063x 2y = 1;



1



Drukarka 3d Tronxy Xy 3 Pro Arm Tmc 300x300x400mm Oficjalne Archiwum Allegro



0 件のコメント:
コメントを投稿